Project Crashing

It is impossible to implement any project in isolation as the events happening around the project affects its progress such as an un-expected calamity or an abrupt economic change, or a nation-wide strike, that may force the project to halt. There can be several reasons which can be either external or internal such as – even if everything goes well, the sponsors may enter the market early.

Here, the project manager plays a key in shortening the project schedule. Some of the commonly used techniques are

Study fast-tracking or shift to an advanced technology.
Add more resources for an early completion. But remember it would invariably result in more costs, therefore a manager must find out if, the costs could be lowered than the crash-cost limit, within the same time-frame.

The process of crashing, aims at reducing time by increasing resources such that the sequence is not disturbed. For instance, if a project involves painting a community hall in 16 days and moving equipments in 8 days, then the total duration becomes 24 days. Here by engaging more workers and excavators, the task can be completed within 12 days and equipments shifted in 4 days, thereby reducing total time to 16 days.

Therefore the main aim of crashing involves time and cost trade off without any change in sequence of activities or task-dependencies. Where on the other hand, fast tracking does not increase costs but increases the risk as many jobs are done in parallel rather sequentially.

Concept of Crashing

In order to assist the manager, two types of networks are defined – First type of network represents normal conditions and the other type of network represents crash conditions. Time and costs are calculated for each activity under both scenarios.

Illustration

In the above table activity ‘A’, shows can be completed within 5 days at a total cost of Rs.5,000. It may be possible to reduce the time to three days by either of the three ways

putting in more resources
allowing overtime-premium
introducing incentives to increase productivity.

Now there are three consideration in time reduction,

Time can only be reduced if we reduce duration of any activity on the critical path. For instance, if we reduce A by 2 days, the total time would be reduced to 15 days. But if we reduce B by 2 days, total time would still remain the same i.e. 17 days.
In this illustration, both A and C can be crashed since both are on the Critical Path. Therefore, we should crash that activity which is cheaper. Incremental Cost for crashing A is Rs.1,250 (difference in Costs divided by difference in Time). The same cost in case of C would be Rs.500 per day. Obviously, we would prefer to reduce C by permissible time of 8 days.

After crashing any activity, we would re-draw the network and re-assess the situation. There are many paths in a network. When we crash activities on the Critical Path, another path may become critical as will be observed later in this hub.

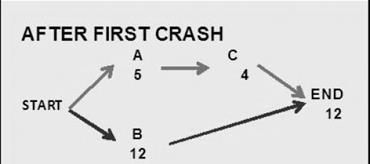
FIRST CRASH

As per the given information above, we can crash C by 8 days for which would have to incur an extra Rs.4,000 (8 days @ Rs.500 per day). After the crash, we re-draw the network.

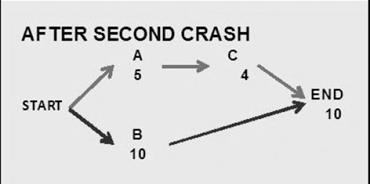
SECOND CRASH

A quick view of the the revised network reveals that total time under the path A- C has reduced to 9 but B, on the other path, has time duration of 12 days. As critical path is the longest path, total time duration has only reduced to 12. Since our crash time is 10 days, we would now reduce B by two days.

UN-CRASHING

In case due to technological reasons, it is not possible to shorten the duration below the crash limit of 10 days even by spending more money or resources. Under such a situation we can review the situation and reduce the crash cost to some extent. This is done through un-crashing.

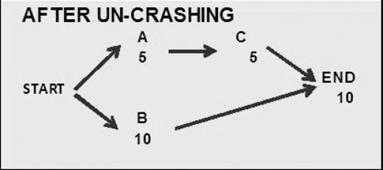
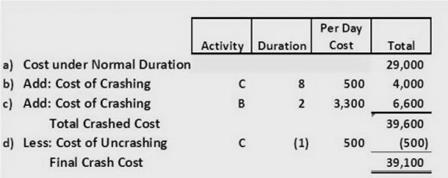
Note, after the second crash, we observed that path A and C had a duration of 9 days. So if we can increase any activity by one day, we would still be under the permissible limit of 10 days. According to the prescribed rules, an activity with the highest per-day cost should be un-crashed. In this case, however, only C can be uncrashed for one day as the other A has never been crashed.

This would lead to making all paths as critical paths such that Total cost would now be Rs.39,100.

Points to be considered for splitting a task

Task considered for splitting should be on the critical path else the overall project duration cannot be reduced.
Tasks after splitting should not all run in series/parallel.
Tasks after splitting cannot use the same resource at the same time.

Illustration

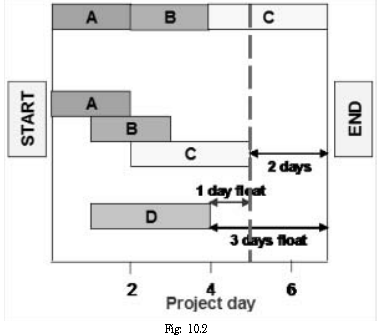
In the above illustration we have single task shown as A B, and C in the block at the top. Note single task is also critical as it goes from beginning to end. We then split these into the individual tasks A B, and C. After considering each activity very carefully we then decide that the task B can start one day after task A starts. Similarly, task C begins one day after the start of task B. So, if we adopt this new structure then the critical path still ends at the end of task C but it is now shortened by 2 days. Where the durations of tasks A B, and C have not changed. Now, within the schedule we have task D as well where the task originally had a total float of 3 days. But due to the reduction in the overall project time its float has been reduced to 1 day.

Remember, when the schedule is more complex one need to take other aspects into account as well. So the original critical path will be shortened but there is no guarantee that it will remain the critical path. Certainly, if the float of a task is less than the original critical path reduction that task will become critical.

For instance,

A task Z has total float = 16 days

Project reduction = 20 days

The total float of task Z = 16 – 20 days = 0 days

Note, the float cannot be -4 days.

Once a task gets to total float as zero then it is on the critical path.

Critical Path

Remember when dealing with critical paths we may have more than one critical path. So if there is more than one critical path and we carry out splitting the task only on one task of one of the critical paths then the overall project duration will not change as it would be controlled by the remaining critical path. So in such a case if you want to reduce the overall project you must carry out task splitting on two tasks i.e., one from each critical path.

So the path that is shortened the least will remain as the critical path. Also you will need to calculate the critical path again before deciding on the next pair of tasks to split. Remember, when you have to split any tasks, specifically in a pair in two critical paths so make sure that the 3-point rule considered above is not broken before going through the cycle again.

Criteria for Selecting Activities to Crash

Should be on the Critical Path: If you are crashing any non-critical activities that already have slack only buys more slack but does not shorten the project duration. Only critical path activities drive the project and crashing them help reduce the project duration.
Must precede multiple activities: If an activity affects several succeeding activities, then it is a great source to be shortened. When specifically this activity is shortened, it then allows the multiple activities to start.
Should have a long duration: When an activity has a long duration offers then it has more potential time gain from crashing it.
Must have lower cost per period gained: Those activities that cost less to crash are more This may include those requiring lower paid, lower skilled workers or some other resources that are not in use.
Should be early in the project: In the initial stage of the project if you encounter a failure in crashing the activity and it takes longer than planned, then it is still early in the projecte., you still have some recovery time. In addition, demand on resources early in the project should be lower than other times, and should be readily available.
Must be labor-intensive: An activity that is low skill labor intensive, it becomes effortless to add people to help complete the project early on. Any activity that requires high skills for completion require qualified individuals competent of completing the task , which may be hard to find.
Should be subject to common problems: We should aim to choose activities that are prone to higher probability of common problems. So shortening the duration reduces the exposure time and thereby cuts the chances of further having a problem.

Steps in Project Crashing

First step involves, computing the crash cost per time period. In case the crash costs are linear over time then,

Crash cost per period = (Crash cost – Normal cost) / (Normal time – Crash time)

Next find the critical path and identify the critical activities using current activity times
In case there is only one critical path, then choose the activity on this critical path that

3.1 can still be crashed, and

3.2 has the smallest crash cost per period.