Present (or Discounted) Value

For finding out the present value of a series of cash flows in future, we may ascertain the present value of each cash flow in future with the formula PV = FVn / (1+r)n or FVn [1/ (1+r)n]and add up.

Alternatively the formula PV = FVn(PVIFrn) may be used for each cash flow and added to ascertain the future value. As we know, the present value of a single cash flow in future is

PV = FVn [1 / (1+r)ⁿ]

For a series of cash flows the formula to be expanded as

PV = FV₁ [1 / (1 + r)¹] + FV2[ 1 / (1 + r)²] + …………

……………………………………….…….+ FVn[1 / (1 + r)n]

In case of Annuity,

PV = A[1 / (1 + r)¹] + A[ 1 / (1 + r)²] + ………… …………………………………………….…….+ A[1 / (1 + r)ⁿ]

Since the future cash flow of annuity is same for every period, the formula is,

PVA_n = A{ [1 / (1 + r)¹] + [ 1 / (1 + r)²] + ……… ………………………………………..……….+ [1 / (1+r)ⁿ] }

= A {[ (1 + r)ⁿ – 1] / [(1+r)n X r]} * mathematical derivation is given at the end of the chapter

It will be clearer for you looking at Graphically the Present Value of an annuity of FV, say, of Re. 1 with discounting rate of 10%

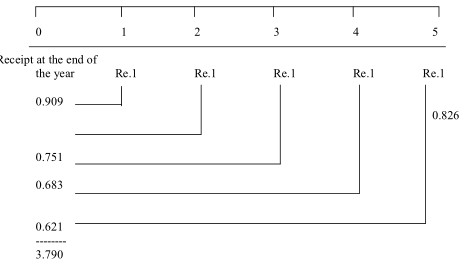
In the above time line discounting is done individually using the formula PV = FVn / (1 + r)^{n .}  Alternatively, table for Present Value Discounting Factor of an Annuity (PVDFA) may be referred  to replace the term [ (1 + r)ⁿ – 1] / [(1+r)ⁿ  X  r] and calculate.

Perpetuities

Perpetuity is an Ordinary annuity whose cash flows continue indefinitely.

This special type of annuity will have to be determined in case of irredeemable preference shares without maturity where preference dividend is expected to be paid perpetually.   Looking at the formula for PVAn i.e. A {[ (1 + r)n – 1] / [(1+r)ⁿ  X  r], this can be rewritten as

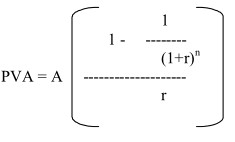
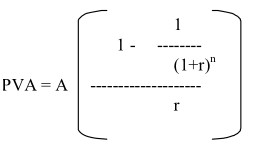
Since any denominator of infinite nature, including any term raised to the power infinity, is zero,

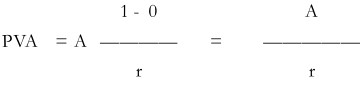
Present Value of Perpetuity

The formula for the PV of perpetuity is derived from the PV of an ordinary annuity, which at N = infinity, and assuming interest rates are positive, simplifies to.

PV of a perpetuity = annuity payment A Interest rate r

Therefore, a perpetuity paying Rs. 1,000 annually at an interest rate of 8% would be worth.

PV = A/R

= Rs. 1000/0.08

= Rs. 12,500

Annuity Due

A series of equal cash flows starting at the beginning of each period is called an Annuity Due.

While calculating future value, Annuity due is simply equal to the future value of a comparable three year ordinary annuity compounded for one more period. This is because last cash flow also accrues interest i.e. for one year., since the cash flow is in the beginning of the year.

Using the formula for future value and providing interest for last cash flow the formula for Annuity Due for future value is

A {[ (1 +r)ⁿ – 1] / r} (1+r)

Alternatively, the Future / Compound Value of Annuity Due may be ascertained by using the Future Value Interest Factor table as below

FVAD_n = A (FVIFA_rn) (1+r)

Similarly Present Value of Annuity Due can be calculated. Since the first cash flow is immediate at the beginning, the Present Value is equal to absolute value and hence the cash flow series will be as below:

PVADn = A {1+[1 / (1 + r)¹] + [1 / (1 + r)²] +

……………….+ [1 / (1+r)^n-1 }

A {[(1 + r)n – 1] / [(1+r)ⁿ     X r]} (1+r) * mathematical derivation is given at the end of the chapter