Modern Portfolio Theory (MPT) refers to the design of optimal portfolios and its implication for asset pricing. It has undergone tremendous development since Markowitz (1952) first laid out the initial mean-variance framework.
A Markowitz portfolio model is one where no added diversification can lower the portfolio’s risk for a given return expectation (alternately, no additional expected return can be gained without increasing the risk of the portfolio). The Markowitz Efficient Frontier is the set of all portfolios of which expected returns reach the maximum given a certain level of risk.
The Markowitz Efficient Frontier
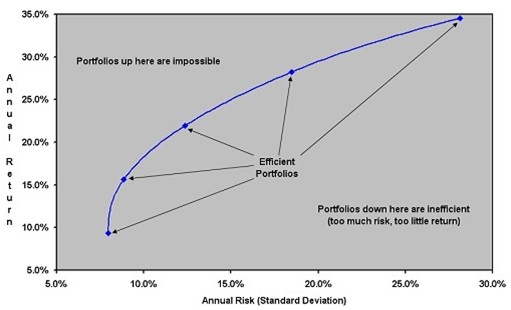
Every possible asset combination can be plotted in risk-return space, and the collection of all such possible portfolios defines a region in this space. The line along the upper edge of this region is known as the efficient frontier. Combinations along this line represent portfolios (explicitly excluding the risk-free alternative) for which there is lowest risk for a given level of return.
Conversely, for a given amount of risk, the portfolio lying on the efficient frontier represents the combination offering the best possible return. Mathematically the efficient frontier is the intersection of the set of portfolios with minimum variance and the set of portfolios with maximum return.
The Markowitz model is based on several assumptions concerning the behavior of investors and financial markets.
- A probability distribution of possible returns over some holding period can be estimated by investors.
- Investors have single-period utility functions in which they maximize utility within the framework of diminishing marginal utility of wealth.
- Variability about the possible values of return is used by investors to measure risk.
- Investors care only about the means and variance of the returns of their portfolios over a particular period.
- Expected return and risk as used by investors are measured by the first two moments of the probability distribution of returns-expected value and variance.
- Return is desirable; risk is to be avoided.
- Financial markets are frictionless.
Markowitz model assumes that investors are risk averse. This means that given two assets that offer the same expected return, investors will prefer the less risky one. Thus, an investor will take on increased risk only if compensated by higher expected returns. Conversely, an investor who wants higher returns must accept more risk. The exact trade-off will differ by investor based on individual risk aversion characteristics. The implication is that a rational investor will not invest in a portfolio if a second portfolio exists with a more favorable risk-return profile – i.e., if for that level of risk an alternative portfolio exists which has better expected returns.
Using risk tolerance, one can classify investors into three types: risk-neutral, risk-averse, and risk-lover. Risk-neutral investor’s do not require the risk premium for risk investments; they judge risky prospects solely by their expected rates of return. Risk-averse investors are willing to consider only risk-free or speculative prospects with positive premium; they make investment according the risk-return trade-off. A risk-lover is willing to engage in fair games and gambles; this investor adjusts the expected return upward to take into account the ’fun’ of confronting the prospect’s risk.
Markowitz’s Portfolio Theory (MPT) is typically applied to assets/portfolios whose return probability distributions approximate to a Normal distribution. Although this approximation is not strictly correct for Hedge Funds, it is still a workable risk model. In fact Fung and Hsieh in [9] apply it to rank Hedge Fund performances. Markowitz in assumes a portfolio’s expected return is equal to the weighted aver-age of the assets’ expected returns and that portfolio risk is equal to the variance of these returns (or in other words the portfolio return’s variance).
MPT also introduces the idea of an efficient frontier. For a given set of funds or assets available to invest in, an upper concave boundary exists on the maximum port- folio returns possible as risk or variance increases. Furthermore this concave relation between risk and return incorporates the theory of expected utility concavely increasing with risk.
Notice that MPT shows that some funds can perform lower than the risk free rate.
Naturally one wishes to choose the market portfolio which maximises return for a given level of risk/volatility as shown.
Apply for Hedge Fund Certification!
https://www.vskills.in/certification/certified-hedge-fund-manager

