Analysis of Variance (ANOVA)

 

Analysis of Variance

ANOVA is used to determine if the average of a group of data is different than the average of other (multiple) groups of data. This tool is used in the ‘Analysis’ phase of a DMAIC Six Sigma project.

The statistical method is applied to test hypotheses among means from several populations. It assumes the sampled populations are normally distributed.

The Y-data is variable type of data (such as time).

The X-data is attribute data (such as appraiser name).

ANOVA is a hypothesis test for means (not median or mode) and usually is applied for testing >2 means. Use 1 sample t or 2 sample t test for one or two means-testing respectively.

It uses two components of variance and the F test to test the two components:

• BETWEEN sample variance
• WITHIN sample varianceBETWEEN sample variance is a study of the variation among all the samples usually due to process difference or factor changesWITHIN sample variance explains the variation within each sample itself (look at a Box Plot of one data set to graphically comprehend this – the tip of one whisker to another)ANOVA shows the right results if the means of several populations are statistically different or equal. It also computes a lot of other valuable insight that can help steer a GB/BB in clearer direction. A statistical difference is found when the difference BETWEEN samples is large enough “relative to the difference WITHIN the samples.

ANOVA Jargon

• Factor (Process Input Variable – PIV, x): A controlled or uncontrolled variable whose influence is being evaluated.
• Factor Level (+1,-1,Hi,Low,+,-,A,B,): Factor setting.
• Response(Process Output Variable – POV, y): The output of the process.
• Inference Space: Range of the factors being evaluated.
• Fit: Predicted value of the POV (y) with a specified setting of factors.
• Residual: Difference from a FIT (predicted output) from the actual experimental output.

One-Way ANOVA Example

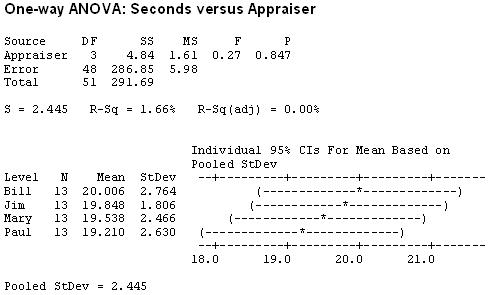
• Determine if there is a significant difference in two or more appraisers. The results of a mock study where four appraisers were timed to make an inspection decision on 13 widgets.
• All other criteria are equal.
• Since TIME is the only factor, this is a One-Factor or One-Way ANOVA. There are four levels that are controlled in the experiment, one being each appraiser.
• The first step is to create the test. In general, if the P-value is lower than the alpha-risk then the alternate hypothesis is inferred (reject the null).

Hypothesis Test:

Null Hypothesis: Population means of the different appraisers are equal.
Alternate Hypothesis: One of the means is not the same

• There are 51 Degrees of Freedom computed from (13*4)-1.
• Using a One-Way test with alpha at 0.05,the P-value is well above 0.05 at 0.847, f-statistic, and heavily overlapping confidence intervals are all evidence that there is no difference among any pairs or combinations of them.
• It is concluded that there is not a statistical difference between any of the appraisers.
• If the P was <0.05, then at least one group of data is different than at least one other group.
• The low F-value of 0.27 says the variation within the appraisers is greater than the variation between them. The F-critical value is 2.81.
• It is advisable to a project manager to re-running (depending on cost and time) the trial with a larger sample size and additional appraiser training to reduce the variation within each one. The variation is fairly consistent among each of them so it appears there is a systemic issue.

Two-Way ANOVA

Other factors can be added to this type of test and get more complicated but most statistical software programs can run Two-Way and Three-Way ANOVA.

Two-Way Hypothesis Tests:

Null Hypothesis: There is no difference in the means of the 1st factor
Null Hypothesis: There is no difference in means of the 2nd factor
Null Hypothesis: There is no interaction between the two factors

Alternate Hypothesis: Means are not equal among the levels of the 1st factor
Alternate Hypothesis: Means are not equal among the levels of the 2nd factor
Alternate Hypothesis: There is an interaction between the two factors

Go back to Tutorial                                                                                Go to Home Page